The rotational movement of bodies is angular velocity. It calculates how fast they go around a point of rotation. There are two kinds of rotations to think about. The first describes the motion of an object’s centre of mass around a defined point in space, known as an origin. Planets orbiting the Sun are two examples, as is a car exiting the motorway. Angular velocity, abbreviated as AV.

The second, known as the spin, depicts the rotation of the body around its own centre of mass. As a result, the quantum attribute of particles is also popular as spin. You’ve most likely seen a basketball player spin a ball on his finger. In general, the faster the movement, the greater the AV. This formula is the subject of this article. So, keep reading to learn more about it.

Angular velocity formula

The AV formula is ω = 2 pi N over 60 rad per second. We already know that the velocity formula in linear motion is the general formula; velocity is just the distance upon the time. If your heart beats twice in one second, your body’s linear velocity is two minutes per second. Assume we apply this method to angular motion as well, resulting in the angular displacement unit per second time. So, if your body is rotating, let N be the body’s rotational speed. The notation rpm denoted rotations per minute. We must divide by 60 if we want per second.

Read Also: Tangential velocity-meaning, formula, vector

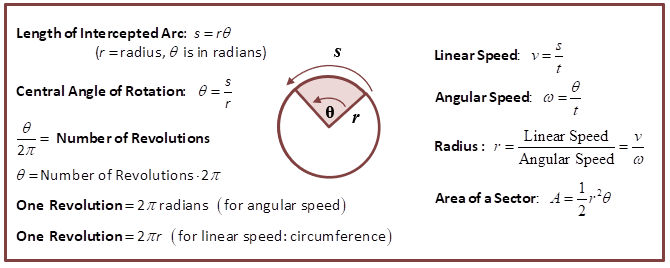
Because one minute comprises 60 seconds, and rotations per minute, translated to seconds by dividing by 60. We know that the body turns one complete rotation, encompassing 360 degrees, which is equivalent to 2 pi radians. If you take one minute, it will be N revolutions. N revolution equals 2 pi upon N radians. Then divide one second by 60 radians per second. So this is 2 pi N at 60 radians per second. We found the AV formula, ω = 2 pi N divided by 60.

ω= 2πN/60 rad/s

Because an object’s angular displacement with respect to time is represented by its AV. So, ω= θ/t denoted AV. Where is the angular displacement, t is the time change, and is the AV. Positive AV indicates clockwise rotation, and negative AV indicates counterclockwise rotation.

Angular velocity formula average

The average angular velocity of a rotating rigid body is the ratio of angular displacement to time interval.

ω average= delta θ/delta t

=θ 2 – θ 1/ t 1 – t 2

Angular velocity formula Instantaneous

The instantaneous angular velocity is the limit of the average angular velocity as the time interval approaches zero.

ω ins = limit delta t tends to 0 delta θ /delta t

=d θ/ dt

Angular Velocity Formula rpm

Revolutions per minute (rpm) and angular velocity are two measures of how fast one point rotates around another. These were used to tackle physics, mechanical engineering, and computer programming problems. rpm and AV are sometimes used interchangeably in engineering simulations and video games to simulate pulleys spinning and wheels rolling.

The pace at which a circular object, like a wheel, spins is referred to as its angular velocity. Because a circle has 360 degrees, a wheel that completes one full rotation around its centre in one second has an angular velocity of 360 degrees per second.

Because it takes 60 seconds for a clock’s second hand to complete one full circuit around its centre, it has an AV of 360 degrees every 60 seconds, or 6 degrees every second.

The pace at which a circular item, such as a wheel, rotates is also described in revolutions per minute. Because one revolution equals one full rotation or spin around a centre point. A wheel that completes one complete turn around its centre in one minute to rotate at one revolution per minute, or 1 rpm.

The rotation rate of a clock is one revolution per minute, or one rpm, since it takes one minute for the second hand of a clock to make one complete turn around its centre.

Angular velocity to rpm conversion

One rotation is 360 degrees, and one minute is 60 seconds. AV in degrees per second converted to rotations per minute by dividing the angular velocity by one-sixth. Because 1/6 multiplied by 6 = 1, the rpm is 1 revolution per minute if the AV is 6 degrees per second.

Angular velocity formula in terms of linear velocity

Let v signify linear velocity and angular velocity, respectively. A body goes on a circular path with radius r at first, and then its position changes from p to q. As a result, it is created in the centre. Angular displacement, on the other hand, is represented by Angular displacement = l divided by r, as we know. Then we may enter l equals r into. Now we must differentiate between the two sides in terms of time t.

As a consequence, dl/dt equal to r can be converted to d/dt where r is constant. After that, dl/dt will be v and d/dt will be. Angular displacement, on the other hand, is now AV, and linear velocity is the rate of change of linear displacement over time. This is a scalar form, whereas v = ω  into r is a vector form. Now, v stands for linear velocity, angular velocity, and r stands for radius vector or position vector.

Then, v= ⍵ ⨯ r.

Angular velocity formula in gravitation

The angular velocity formula is ω= 2 pi upon T. We’ll suppose it’s spinning in a circle. T, on the other hand, is just the length of time it takes to spin. However, we know that one Earth revolution takes 24 hours. We must, however, convert the hour to the minute. As a result, we must convert 24 hours (24 = 24) to 3600. On 86400, is now equal to 2 pi.

ω=dθ/dt

Here,dθ=2πRadians

dt = 24Hours

Now, The SI unit of angular velocity is rad/sec

so we need to convert our time to seconds

Next, 1 hour = 3600 sec

⇒24 hours

=24×3600

=24×3600 sec

Now, ω=2π/24×3600 rad/sec

⇒ω=7.29×10^−5 rad/sec

Angular velocity formula in vector form

The magnitude of the angular velocity vector is just the rate at which it spins – the angle swept out per unit time. Angular velocity is denoted by the Greek letter (let’s call it “w” instead because I don’t have a Greek font!) and is measured in radians per second.

The direction of the angular velocity vector, on the other hand, is difficult to “get.” Because each of the rotating object’s various pieces moves in a different direction. So, despite the fact that nothing moves in that direction, the approach for identifying the direction of the angular velocity vector is to allow it to be along the axis of rotation, with the direction of that vector following what is known as the “right-hand rule.”

Curl your right hand’s fingers with the sense of rotation, and your right thumb will point in the direction of the vector. If the disc rotates as shown in the illustration, the angular velocity vector should be upward and parallel to the axis of rotation, according to the right-hand rule.

Take a look at the prior graphic of the disc rolling from left to right to see whether this is clear. Although it’s unclear whether this is clockwise or counterclockwise depending on which side of the disc you’re looking at, curling your right-hand fingers with the same feeling of rotation as the disc, your right thumb points into the page – regardless of whose side you’re looking at.

That is, the angular velocity vector would have a magnitude equal to the rotation rate of the disc and would be directed according to the right-hand rule.

Angular velocity formula circular motion

Circular motion is the movement of an object in a circular path. Circular motion can be seen in a race car travelling around a circular curve, a toy attached to a thread swinging in a circle around your head, or the circular loop-the-loop on a roller coaster. The rotation of an item on an axis that passes through its centre of mass, such as the Earth rotating on its axis, a wheel turning on its axle, the spin of a tornado on its path of devastation, or a figure skater spinning during an Olympic performance, is referred to as spin.

Objects can spin while moving in a circular motion, such as the Earth revolving on its axis while orbiting the Sun, but we will examine these two movements separately.

ω = r v / |r|^2, where all of these variables are vectors and |r| represents the absolute value of the radius. In actuality, the angular velocity is a pseudovector with a perpendicular direction to the plane of rotational movement.

Angular velocity formula dimension

The dimensional formula for angular velocity is ω = angular displacement upon time. The radian per second is currently the SI unit for angular velocity. is no longer a dimensionless quantity. If a particle has a radius of r and a beginning point of p, the particle’s position will be p after some time. The angular displacement formula is arc upon radius. The symbol s indicated the arc length. As a consequence, s divided by r Equals s Nows measures the intern of the metre, and r likewise measures the intern of the metre. Then, as a result, both metres will be cancelled, resulting in a score of 1.

As a consequence, both metres are cancelled, providing a value of 1. The new dimensions of angular displacement are M power zero, L power zero, and T power zero. It is a non-dimensional quantity.

As a result, the angular displacement is stated as 1 upon t, and because t is just time, the SI unit of time is the second. When the second is expressed in terms of the dimension T, ω = 1. T is now the numerator, while T to the power minus 1 is the denominator. In the dimensional formula, angular velocity, expressed as M to the power absent, L to the power absent, and T to the power minus 1.

Proof

Angular Velocity = Angular displacement × [Time]-1…(1)

Now, the dimensional formula of Angular displacement = [M^0 L^0 T^0]….(2)

And, the dimensions of time = [M^0 L^0 T^1]……(3)

Substituting equations (2) and (3) into equation (1) yields,

Next, angular Velocity = Angular displacement × [Time]-1

Or, v = [M^0 L^0 T^0] × [M^0 L^0 T^1]-1 = [M^0 L^0 T^-1]

The angular velocity, on the other hand, is dimensionally represented as [M^0 L^0 T^-1].

Angular velocity formula with radius

Assume we have an object moving in a circular motion. As a consequence, the object is travelling in a circular direction that mimics a clockwise circular path from the centre. The item is now revolving at a rate of five revolutions per second. However, radians are simply one type of angle measurement. You may also use degrees per second instead. When we do it in radians, we notice that each revolution is 2 pi radians long. Then, if we complete a circle, we have travelled 2 pi radians. We can conduct some dimensional analysis because each rotation has 2 pi. After that, we multiply 5 times 2 pi to get 10 pi radians per second. It also does dimensional analysis and is logical.

Each revolution is two pi radians if we rotate at five rotations per second.

As a result, we’re travelling at a pace of 10 pi radians per second. So 5 revs per second and 10 pi radians per second are measuring the same thing. In contrast, angular velocity is a measure of how quickly you rotate around a central point. Then there’s angular velocity, which tells us how quickly our angle changes or how quickly the angle changes. Angular velocity, on the other hand, is frequently mistaken for angular speed. Then there’s the fact that it’s a vector quantity, and the fact that the vector is physically bursting out of the page is puzzling. However, it is a fake vector.

As a result, it is a vector quantity, and the direction defines the vector’s orientation in which it spins. When it rotates counterclockwise, for example, there is a vector; the correct angular vector appears on the page.

Result

When the page turns clockwise, the angular velocity vector will show. When considering a two-dimensional plane, an angular velocity may be thought of as a pseudo-scalar. However, if we designate which way it rotates, we may include it as a scalar number. We can refer to 10 pi radians per second as angular velocity. And an ω commonly represents this. Angular velocity, on the other hand, is a change in angle upon time. So, based on the computation results, we can assume that is equal to the speed for which we are using v upon the radius.

Angular velocity formula with frequency

The angular velocity of an item is the rate at which its angular position changes with respect to time. The following formula expressed an important relationship between angular velocity and frequency. Angular velocity, calculated by multiplying frequency by the constant 2pi. The constant 2 pi comes from the fact that one rotation per second equals two pi radians per second. In other words, an item rotates at a rate of 2 pi radians per second when it completes one full rotation per second. Period, which is the amount of time in seconds necessary for an item to complete one full rotation, can also be used to describe angular velocity.

1: Determine the angular frequency of an oscillating object having a 30 second time period.

Solution: As stated previously

T = 30 seconds

Using the formula,

ω=2πT

Changing the values,

ω=2π30

= 360°30

ω=12°sec−1

As a result, the angular frequency is 12°/sec.

Angular velocity formula examples

Q1) Determine the angular velocity of a 0.500 m radius bus tyre travelling at 10.0 m/s (about 36 km/h).

Solution:

Given:

v= 10.0 m/s

r= 0.500m

We are aware that

v= r ω

or

ω = v/r

We obtain by substituting the supplied numbers in the preceding equation:

ω= 10.0 m/s /0.500m = 20 rads^-1

When we cancel out the units in the previous computation, we obtain 20.0/s. However, the angular velocity must be measured in rad/s. Because radians are unitless, we can simply plug them into the angular velocity solution.

Q2) On the highway, an automobile wheel with a radius of 20 inches revolves at eight rotations per second. What is the tire’s angular velocity?

Solution:

The angular velocity may be calculated using the following equation:

ω= θ/t

We multiply by two since a full rotation (360°) equals two because the automobile has completed eight rotations each second.

We obtain by substituting the numbers in the equation,

ω= 16 pi radians/1 s = 16 pi rads^-1

As a result, the tire’s angular velocity is

16 pi rads^-1

Some frequently asked questions

How do you calculate angular velocity?

The rate of change of an object’s position angle with respect to time is angular velocity, therefore w = theta / t, where w = angular velocity, theta = position angle, and t = time.

What is the angular velocity we have?

The rate of change of an angle is angular velocity ω. Then, ω, represented in symbols as ω=ΔθΔt ω = Δ θ Δ t, where an angular rotation Δθ occurs in time Δt. The angular velocity increases as the rotation angle increases in a certain length of time. Radians per second (rad/s) are the units for angular velocity.

What is the formula for angular velocity and angular acceleration?

The velocity changes with time in non-uniform circular motion, and the rate of change in angular velocity (i.e. angular acceleration) is  α=ΔωΔt α = Δ ω Δ t.

How do you calculate velocity?

Velocity (v) is a vector quantity that represents displacement (or change in position, Δs) over time (Δt). The equation v = Δs/Δt expressed it.  Speed (or rate, r) is a scalar number. It represents the distance travelled (d) divided by the change in time Δt) by the equation r = d/Δt.

How do you find angular velocity with revolutions?

Because one revolution is 360 degrees and there are 60 seconds each minute, revolutions per minute may be translated to angular velocity in degrees per second by multiplying the rpm by 6. If the rpm is one revolution per minute, the angular velocity in degrees per second is six, since six multiplied by one equals six.

What is an example of an angular velocity example?

It is less frequent than linear velocity since it only applies to things travelling in a circular route. An example of angular velocity is a roulette ball on a roulette wheel, a race vehicle on a circular circuit, and a Ferris wheel.

How do you find angular velocity with radius and time?

If v represents a spinning object’s linear speed, r its radius, and ω its angular velocity in radians per unit of time, then v = rω. This is a very important formula since it connects these three quantities such that if we know two, we can always determine the third.

What is the formula for linear velocity?

We can determine the linear velocity of an item by the distance it travels in relation to the time it takes. v = x/t is the linear equation or linear velocity formula. Where v denotes linear velocity.

How do we calculate torque?

Calculate the distance, r, between the pivot point and the place where the force is applicable. Determine the angle θ formed using the applied force’s direction and the vector connecting the point at which the force is applicable to the pivot point. Torque, calculated by multiplying r by F and sin θ.

How do you calculate change in velocity?

• The rate of change of velocity is acceleration. It is the rate at which velocity varies per unit of time.
• The velocity change estimated using the equation: velocity change = final velocity – initial velocity.