This equilibrium constant calculator will assist you in understanding reversible chemical processes, which are reactions that occur in both the forward and backward directions at the same time. After a particular length of time, an ⇌ is reached, which means that the rate at which reactants are converted into products is the same as the rate at which products are converted back into reactants. The response is deemed steady at this stage. The reaction quotient should remain constant in order to determine the state of this ⇌. You may use this tool to compute the value of an ⇌ constant for a reaction.
This is one of the most effective ⇌ constant calculators for finding the ⇌ constant Kp for the provided chemical equation using partial pressure. You may use this Partial pressure calculator to enter your chemical equation and get the balanced equation. The balanced equation’s components, supplied as separate tables for the reactants and products. Finally, the ⇌ constant (kp) is given in atm units.
In this article, we are talking about this calculator. So, keep reading to know more about it.
Equilibrium calculator economics
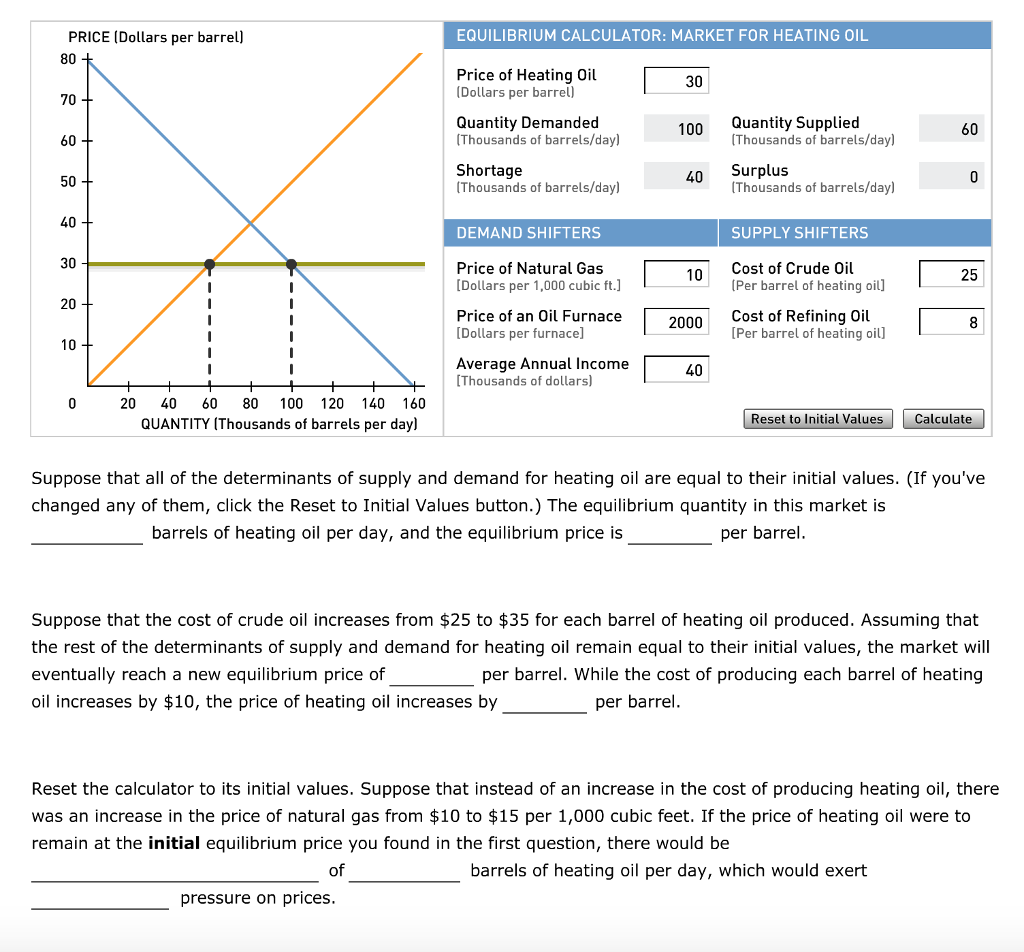
The point at where the cost of a product and the demand for that product intersect, resulting in a price compromise, referred to as the ⇌ price. There is a balance between customers purchasing the goods and firms delivering the product at the ⇌ price. When a product is in market ⇌, there is no pressure from the consumer or the corporation to raise or lower the price, and supply and demand amounts are balanced. When the market is in ⇌, the price of a commodity remains steady, rises when there is a scarcity, and falls when there is a surplus.
In other words, if you had a graph of supply and demand for a commodity, the point where the supply curve crosses the demand curve is the point of ⇌. At this time, both customers and producers have agreed on the amount and price of the product. If either the amount or the cost of a product changes, the market for that commodity no longer has an ⇌ quantity or an ⇌ price.
Equilibrium calculator Formula
The ⇌ price of a product may be calculated theoretically, assuming that the amount demanded equals the quantity provided. You may use linear algebraic equations to locate the intersection of a product’s supply and demand lines on a graph. This point of intersection is the ⇌ pricing formula, which equalizes the supply and demand functions. These three formulae are as follows:
The linear supply function is as follows:
Qs = yP + x
Where:
Qs denotes the quantity delivered.
X stands for quantity.
P stands for price.

The linear demand function is as follows:
Qd = x plus yP
Where:
Qd denotes the quantity of demand.
X stands for quantity.
P stands for price.
The ⇌ price is the price at which the two are equal to each other:
Qd = Qs
Equilibrium calculator supply and demand
Supply and demand is an economic model of price setting in a market in microeconomics. It holds that in a competitive market, the unit price of a specific good or other traded item, such as labor or liquid financial assets, will fluctuate until it settles at a point where the quantity demanded (at the current price) equals the quantity supplied (at the current price), resulting in an economic ⇌ for price and quantity transacted.
- For quantity, use the supply function. The supply formula, Qs = x + yP, is used to find the supply line algebraically or on a graph.
- For quantity, use the demand function.
- Set the prices of the two amounts to be the same.
- Determine the ⇌ price.
Equilibrium calculator chemistry
Chemical ⇌ is the condition of a chemical process in which both the reactants and products are present in concentrations that have no further tendency to vary with time, resulting in no apparent change in the system’s characteristics. This is the condition that occurs when the forward reaction and the reverse reaction both proceed at the same pace. The reaction rates of forward and backward reactions are not always zero, but they are always equal. As a result, no net changes in the concentrations of the reactants and products occur. This is referred to as dynamic ⇌.
Following the discussion of the fundamental ideas of chemical equilibria in the preceding sections of this chapter, the last section will illustrate the more practical element of applying these concepts and proper mathematical methodologies to conduct various ⇌ calculations. These sorts of calculations are critical in many fields of research and technology, such as pharmaceutical product development and dosage. After a medicine is consumed or administered, it normally through a number of chemical equilibria that influence its eventual concentration in the bodily system of interest. To calculate a dose level that would produce the desired therapeutic effect, knowledge of the quantitative elements of these equilibria is essential.
Equilibrium calculator Reaction
Many of the relevant ⇌ calculations that will be taught here need the inclusion of terms that indicate changes in reactant and product concentrations. These terms are obtained from the reaction’s stoichiometry, as demonstrated by ammonia decomposition:
2NH3(g)⇌N2(g)+3H2(g)
As previously demonstrated in this chapter, this ⇌ may be achieved within a sealed container containing either simply NH3 or a combination of any two of the three chemical species participating in the ⇌. A reaction mixture, regardless of its original composition, will exhibit the same correlations between changes in the concentrations of the three species involved, as stipulated by reaction stoichiometry (see also the related content on expressing reaction rates in the chapter on kinetics). For instance, if the nitrogen content rises by a certain amount x,
Δ[N2]=+x
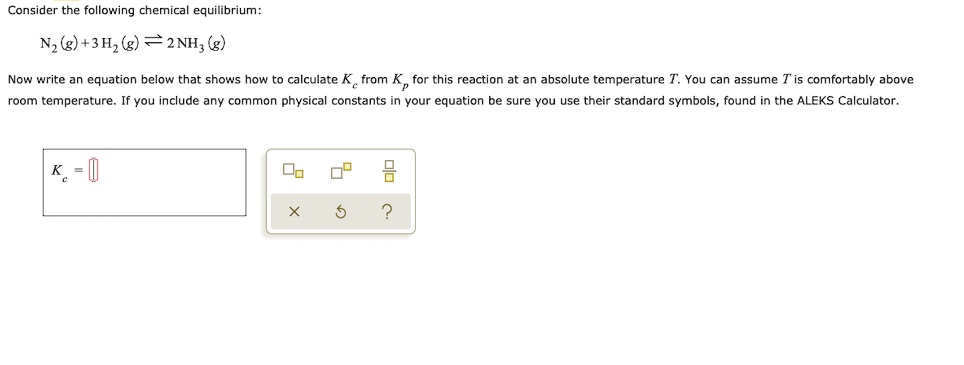
The equivalent changes in concentrations of the other species are
Δ[H2]=Δ[N2](3 mol H2/1 mol N2)=+3x
Δ[NH3]=Δ[N2](2 ml NH3/1 mol N2)=−2x, where the negative sign implies a drop in concentration.
Equilibrium calculator Example
What is the ⇌ constant of the reaction 2SO2 + O2⇌ 2SO3?
Answer:
The given chemical reaction is 2SO2 + O2 2SO3.
Then, K = 2[SO3]2/(2[SO2]2 * [O2]) is the ⇌ constant equation for this reaction.
Then, the concentrations of reactants and products in the state of ⇌ are as follows:
0.03 mol/L SO2
Also, 0.035 mol/L O2
Then, 0.5 mol/L SO3
Also, K = (2*0.5)2/((2*0.03)2*0.035)
Then, = (0.0036 * 0.035)/(0.0036 * 0.035)
Then, =1/0.000126
Atlast, =7936.5
When K is greater than one, the ⇌ favors the products.
Equilibrium calculator math
The ⇌ price of a product may be calculated using the supply function, demand function, and ⇌ pricing formula, which puts the first two functions equal to each other. These instructions will utilize a fictitious corporation that sells hats to help you learn how to use the formula. Here’s how to calculate a product’s ⇌ price:
Equilibrium calculator for quantity, use the supply function.
To get the supply line algebraically or on a graph, use the supply formula, Qs = x + yP. In this equation, Qs denotes the number of delivered hats, x is the amount, and P denotes the hat price in dollars. Assume there is a demand for 100 hats at a price of $1.
100 + 1P = Qs
Equilibrium calculator for quantity, use the demand function.
To find the demand line algebraically or on a graph, use the demand formula, Qd = x + yP. In this equation, Qd is the number of hats required, x denotes the quantity, and P denotes the price of hats in dollars. Assume that the provider can deliver 400 hats at a cost of $5.00 per hat.
400 + 5P = Qd
Set the prices of the two amounts to be the same.
To obtain the equilibrium price, you must first put the supply function equal to the demand function, so that Qs = Qd. This is how it appears for this problem:
Qd = Qs
100 + 1P equals 400 + 5P
Determine the equilibrium price.
To find P, or the price, use the basic laws of algebraic equations. The steps are as follows:
100 + 1P equals 400 + 5P (subtract 1P from both sides of the equation)
100 x 400 x 4P (subtract 400 on both sides of the equation)
-300 x 4P = (divide by 4 on both sides of the equation)
P = -75
P = $-0.75
Because this example has a negative equilibrium price, there is a negative slope and a bigger amount required than is available at the equilibrium price. In this case, the corporation has discovered that there is a scarcity of hats. To fulfill demand, they should create additional hats during the following accounting period.
Equilibrium calculator for differential equations
Assume we have a differential equation dy dt=f (t,y). It is sometimes possible to identify instantaneous answers just by analyzing the differential equation.
Take, for example, the differential equation dy dt=2y2+y. One apparent solution to this differential equation is y=0, since if y=0, the differential equation above simplifies to ddt(0)=0, which is correct. Furthermore, if we factor the right hand side of the differential equation shown above, we get:
dy dt=2y(y+12)
As a result, y=12 is also a solution, which you should check. These answers are unique, and we shall name them in the definition below.
Equilibrium Solutions are achieved by setting dy dt=0 and solving for y if dy dt=f(t,y) is a differential equation. Take a look at these samples.
Example 1
Find the equilibrium solutions to the differential equation dy dt=y29.
The preceding differential equation may be expressed as dy dt=(y+3)(y3). As a result, if y=3 or y=3, which are the equilibrium solutions, dy dt=0.
Example 2
Find the equilibrium solutions to the differential equation dy dt=yt 2 yt+y2.
The preceding differential equation may be expressed as dy dt=y(t2-t+y). As a result, one of the equilibrium solutions is y=0. When t2t+y=0, another equilibrium solution is achieved, namely y=tt-2.
Equilibrium calculator statistics
When all of the forces acting on an item are balanced, it is said to be in a state of equilibrium. If the rightward forces are balanced by the leftward forces and the upward forces are balanced by the descending forces, the forces are said to be balanced. This does not necessarily imply that all of the forces are equal to one another. Consider the two objects represented in the force diagram below. The two objects are in equilibrium because the forces acting on them are balanced; nevertheless, the individual forces are not equal. The force of 50 N is not equivalent to the force of 30 N.
Read Also:Everything about Proportion Calculator
When a thing is in equilibrium, its forces are balanced. The crucial term for describing equilibrium conditions is “balanced.” As a result, the net force is 0 and the acceleration is 0 m/s/s. Equilibrium objects must have an acceleration of 0 m/s/s. This is an extension of Newton’s first law of motion. However, an acceleration of 0 m/s/s does not imply that the object is at rest. When an object is in equilibrium, it is either at rest and remaining at rest, or in motion and continuing to move at the same pace and direction.
Equilibrium calculator game theory
Example 1
Two companies are combining to become two divisions of a larger corporation, and they must decide which computer system to utilize. The businesses, I and A, have previously utilized various systems; each likes the system it has previously used. They will both benefit from using the same system rather than continuing to utilize distinct systems.
The following two-player strategy game can be used to mimic this circumstance.
| I | A | |
| I | 2,1 | 0,0 |
| A | 0,0 | 1,2 |
We investigate each action profile in turn to determine the Nash equilibria.
(I,I) Neither player can increase her payoff by taking a different action than the one she is currently taking. As a result, this activity profile represents a Nash equilibrium.
Then, (I,A) By selecting A rather than I, player 1 receives a 1 rather than a 0 reward as a result of player 2’s action. As a result, this action profile does not correspond to a Nash equilibrium. [Player 2 can also boost her payout by selecting I rather than A.]
Then, (A,I) Given player 2’s action, choosing I rather than A results in a reward of 2 rather than 0. As a result, this action profile does not correspond to a Nash equilibrium. [Player 2 can also boost her payout by selecting A rather than I.]
(A,A) Neither player can enhance her payout by doing a different action than the one she is now taking. As a result, this activity profile represents a Nash equilibrium.
We find that there are two Nash equilibria in the game, (I,I) and (A,A).
Example 2
A product’s look must be chosen by both an established corporation and a newcomer to a fixed-size market. Each company can select between two possible product looks, denoted by the letters X and Y. The established manufacturer wishes that the newcomer’s product seem distinct from its own (so that its clients are not enticed to buy the newcomer’s product), but the newcomer prefers that the items look similar.
Then, the following two-player strategy game can be used to mimic this circumstance.
| I | A | |
| I | 2,1 | 1,2 |
| A | 1,2 | 2,1 |
We investigate each action profile in turn to determine the Nash equilibria.
(X,X)Firm 2 may boost its payout from 1 to 2 by selecting action Y instead of action X. As a result, this action profile does not correspond to a Nash equilibrium.
(X,Y) Firm 1 may boost its payout from 1 to 2 by selecting action Y instead of action X. As a result, this action profile does not correspond to a Nash equilibrium.
(Y,X) Firm 1 may boost its payout from 1 to 2 by selecting action X instead of action Y. As a result, this action profile does not correspond to a Nash equilibrium.
(Y,Y) Firm 2 can boost its payout from 1 to 2 by selecting action X instead of action Y. As a result, this action profile does not correspond to a Nash equilibrium.
Equilibrium calculator physics
A reaction’s equilibrium constant is related to all of the species present in the reaction. However, we assume in this calculator that there are a maximum of two primary reactants and two main products. For the speculative reaction:
a[A] + b[B] ⇌ c[C] + d[D]
The following is the formula for the equilibrium constant equation:
([C]c * [D]d)/([B]b * [A]a) = K
The constant K represents two different measures of quantity:
- First, Kc denotes concentration, molarity, and represented in moles per liter (M=mol/L).
- Then, Kp – a function of both reactant and product partial pressures, generally in atmospheres, helpful for gas phase computations.
- Then, If K is greater than one, equilibrium favors the products.
- So, If K1 – equilibrium is in favor of the reactants,
- Then, If K=1, the mixture in equilibrium has comparable quantities of both products and reactants.
Equilibrium calculator example
The following is an example of determining the equilibrium pricing for organic pineapples sold at a fruit stand:
The fruit stand formerly sold 500 pineapples for $4.00 each month, but now wants to sell 900 pineapples for $3.00 each month. The fruit stand may use this information to determine if the new supply and demand amounts are at the equilibrium price.
Here, we enter the amount of pineapples delivered as well as the price of those pineapples into the calculation.
500 + 4P Qs = 500 + 4P Qs = 500 Qs = 500 Qs
Then we take the amount of pineapples that have been requested and the price at which the fruit stand is considering selling them.
900 + 3P = Qd

Set the prices of the two amounts to be the same:
Qd = Qs
500 plus 4P equals 900 plus 3P
Take the preceding equation and simplify it:
500 plus 4P equals 900 plus 3P
Then, 500 plus 1P equals 900
Then, 1P equals 400
Also, P equals 400
Then, P equals $4.00
This suggests that the pineapples at the fruit stand have an equilibrium price of $4.00. If the fruit stand drops the price below $4.00, purchasers will desire more pineapples than the fruit stand can sell due to an excess of demand. If the fruit stand raises the price above $4.00, purchasers will demand fewer pineapples than the fruit stand can sell due to an excess of supply. Then, if the fruit stand maintains its pineapple prices at $4.00, there is a balance between supply and demand that benefits both the purchasers and the fruit stand.
Some frequently asked questions
How do you calculate equilibrium?
The following is how to use the equilibrium constant calculator:
- In the input field, enter the reactants, product coefficients, and concentrations.
- To obtain the output, click the “Calculate Equilibrium Constant” button.
- Finally, in the output field, the equilibrium constant for the selected chemical process will be presented.
What is the general formula of equilibrium?
At a particular temperature, Keq is the equilibrium constant. This is popular as the equation of the law of chemical equilibrium. If the concentration of reactants stated as moles/lit at equilibrium, Keq = Kc, and if it is written as partial pressure, Keq = Kp.
How do you solve equilibrium problems?
Make a table with the starting concentrations of all the chemicals in the combination. Fill up the table with the concentration changes (x) and final concentrations. B Write the reaction’s equilibrium constant expression. To solve for x, substitute the known K value and the final concentrations.
What is K in equilibrium?
The equilibrium concentrations of all reactants and products assessed in an equilibrium reaction. The equilibrium constant (K) is a mathematical relationship that demonstrates how the concentrations of the products change when the concentrations of the reactants change.
What is K in a rate law?
The specific rate constant (k) is the proportionality constant that relates the reaction rate to the concentrations of reactants. For each chemical reaction, the rate law and particular rate constant must be established experimentally. The rate constant’s value varies with temperature.
What is an example of equilibrium?
Example 1
Find the equilibrium solutions to the differential equation dy dt=y29.
The preceding differential equation may be expressed as dy dt=(y+3)(y3). As a result, if y=3 or y=3, which are the equilibrium solutions, dy dt=0.
Example 2
Find the equilibrium solutions to the differential equation dy dt=yt 2 yt+y2.
The preceding differential equation is dy dt=y(t2-t+y). As a result, one of the equilibrium solutions is y=0. When t2t+y=0, another equilibrium solution is achieved, namely y=tt-2.
Is equilibrium chemistry easy?
Equilibrium is a simple issue – a huge name, but a simple concept. To begin, when you have a system made up of several molecules, those molecules will occasionally combine. That is the concept of a chemical reaction.
Can K be negative?
Because the concentration of the reactant falls with time, the value of k is negative. A graph representing the concentration of any product as a function of time, on the other hand, is a straight line with a slope of k, a positive number.




