The combine like terms calculator is a free online tool. Also, it can assist in combining like terms in an equation and simplifying it. Moreover, this is a useful tool for solving polynomial equation issues since it simplifies and speeds up the calculating process.

Then, if two words have the same variable portion, we call them “like terms.” For example, 4x and 3x are synonymous, while 4x and 3w are not. In this article, we are talking about this calculator. So, keep reading to know more about it.
Combine Like Terms Calculator with steps
This is a very basic tool for merging similar phrases. Please use the online combining like terms calculator to combine like terms of the algebraic statement as shown below:
- First, navigate to any online combining like terms calculator.
- Then, enter the algebraic expression into the combining like terms calculator’s input box.
- Then, to combine like phrases, click the “Solve” button.
- Atlast, click the “Reset” button to clear the field and start over.
Combine Like Terms Calculator free
So, Combine like terms Calculator is an online app. Also, it allows you to combine like terms to simplify an algebraic statement. Then, we can see both like and unlike words in an algebraic expression. Then, the Combining Like Words Calculator assists you in combining like terms and reducing an algebraic statement. So, by adding or removing two similar words, they can be merged.
Read Also:Rationalize the denominator calculator
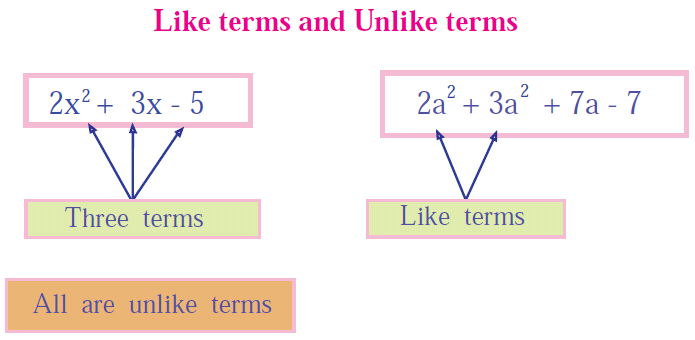
In an equation, similar terms are the terms that have equivalent powers. For example, x^2 and 2x^2 are synonyms. Similarly, 3x^3 and 54x^3 are synonyms. The “Combine Like Terms Calculator” calculator returns 3x^2 + 19 for the problem 2x^2 + 13 + x^2 + 6.
In mathematics, algebraic expressions are expressions that include variables and constants as well as arithmetic operations such as addition, subtraction, multiplication, and division. As an example: 3x +19y = 30 is an algebraic expression since it has three terms: 3x, 19y, and 30. The first two terms are 3x and 19y, with x and y being variables and 30 being a constant. As a result, algebraic terms are discrete parts of an equation separated by plus or minus signs. There are two kinds of algebraic words: like algebraic terms and unlike algebraic terms.
Combine Like Terms Calculator show work
An algebraic expression’s terms are defined as the distinct elements separated by a plus or minus sign. In an algebraic equation, like terms are ones that have the same variables. These variables are then increased to the same power. The coefficients of such words can vary. Unlike terms, on the other hand, contain various variables that can be increased to different exponents. On like terms, addition, subtraction, multiplication, and division can be accomplished. However, in order to combine two words, addition and subtraction cannot be done on them. The following are the procedures for simplifying the algebraic equation by merging like terms:
- If the expression contains brackets, we must open them.
- Put together the phrases that contain the same variables and are raised to the same exponents.
- Add or subtract these comparable phrases based on the sign. As a result, the algebraic equation will be simplified to its most basic form.

Like terms are ones that have the same variables and exponent power. The coefficients of these factors might vary. Algebraic-like words are terms that are related to one another. The algebraic expression’s similar terms can be combined to simplify the equation and deduce the solution in a straightforward manner. For example, this is equivalent to the algebraic statement 8y + 2y, where y is the same variable in the expression and the coefficients differ. To make it more simpler, we may combine the two like words, i.e. 8y + 2y = 10y. As a result, all arithmetic operations, including addition, subtraction, multiplication, and division, can only be done on algebraic expressions.
Combine Like Terms Calculator Examples
Example 1
Combine the similar terms 8x^2 + 6x + 6x^2 + 9 + 8x to simplify the following statement. Use the online combining like phrases calculator to confirm the answer.
Solution:
When we put similar phrases together, we get,
= 8x^2 + 6x^2 + 6x + 8x + 9
By combining similar phrases,
= 14x^2 + 14x + 9
As a result, the sum of similar words Equals 14x^2 + 14x + 9
Example 2
Combine the similar terms 9yx + 10x^2 + 3.5y^2 – (7x + 5x^2 – 2xy + 1.2y^2) to simplify the provided calculation. Use the online combining like phrases calculator to confirm the answer.
Solution:
Taking out the brackets,
= 9yx + 10x^2 + 3.5y^2 – 7x – 5x^2 + 2xy – 1.2y^2 – 7x – 5x^2 + 2xy – 1.2y^2
When we put similar phrases together, we get,
9yx + 2xy + 10x^2 – 5x^2 + 3.5y^2 – 1.2y^2 – 7x
By combining similar phrases,
11xy + 5x^2 + 2.3y^2 – 7x = 11xy + 5x^2 + 2.3y^2 – 7x
As a result, the sum of comparable words is 11xy + 5x^2 + 2.3y^2 – 7x.
Similarly, you may use the online combining like terms calculator to combine the following like phrases:
x^3 + 4yx^2 + 8 + 4y + 8.2x^3 + 5yx^2
7.2x^2 + 6yx^3 +5xz^2 + 5 + 6.3x^2 + 4z^2x
Example 3
Combine similar phrases. What is a more basic version of the expression? -3(-4y + 3) + 7y
Solution:
It is assumed that
-3(-4y + 3) + 7y
Using the distributive multiplicative property
= 12y – 9 + 7y
= 19y – 9
As a result, the simplified version of the statement is 19y – 9.
Example 4
Combine similar phrases. What is a more basic version of the expression? -2(-3y + 4) + 5y
Solution:
It is assumed that
-2(-3y + 4) + 5y
Using the distributive multiplicative property
6y – 8 + 5y
= 11y – 8
As a result, 11y – 8 is the simpler version of the statement.
Combine Like Terms Calculator rules
When you combine related terms to simplify a phrase, certain criteria apply. We’d try to go through all of the operation sequence rules one by one. The acronyms listed below are commonly used in mathematical calculations, therefore it is important to comprehend these terminology. To simplify your algebraic statement, use a Combining like terms calculator.

- PEMDAS is an acronym that stands for Parentheses, Exponent, Multiplication, Division, Addition, and Subtraction.
- BEMDAS: BEMDAS is an acronym that stands for Barentheses, Exponent, Multiplication, Division, and Addition and Subtraction.
- BODMAS: “Brackets, Order, Division and Multiplication, Addition and Subtraction” is an abbreviation for “Brackets, Order, Division and Multiplication, Addition and Subtraction.”
- GEMDAS: “Grouping, Exponents, Division and Multiplication, Addition and Subtraction” is an abbreviation for “Grouping, Exponents, Division and Multiplication, Addition and Subtraction.”
- MDAS is a subset of the acronyms listed above. It is an abbreviation for “Multiplication, Division, Addition, and Subtraction.”
Because these terms are used synonymously, it would be advantageous for us to solve the same algebraic terms by combining similar phrase calculators when combining like terms to generate an equivalent equation.
Associativity of Operators
The left associate operations include multiplication, division, addition, and subtraction. When you solve the four operators listed above, you start from the left side. When you add and subtract like terms, you use the operators associative property. The combine like terms calculator automatically determines whether to utilise the left or right associative attribute.
- X/Y* Z= (X/Y)* Z
- X+Y-Z= (X+Y) -Z
Right-Associative property:
- xyzn = x(y(zn))
- xry(n/z) = xr(y(z/n))
We must first solve the innermost brackets, followed by the inner parentheses or brackets. We utilise the PEMDAS to solve the right associative property, and the numbers may be checked using the combine like terms calculator.
Addition Operations (+) Rules
When combining two similar phrases with the same symbols, preserve the symbols and simplify and combine the terms. The following are some instances of combining similar phrases with addition operations:
| (-)+(-) = (-) | (+)+(+) = (+) |
| (-15x)+(-5x) = (-20x) | (+12x)+(+8x) = (+20x) |
If the Symbols vary, then remove the words while keeping the symbols of the bigger term.
| (-Large)+(+Small) = (-) | (-Small)+(+Large) = (+) |
| (-15x)+(+5x) = (-10x) | (-6y)+(+8y) = (+2y) |
Subtraction Operations (-) Rules
Keep the first term’s sign and then modify all the other signs before applying the same addition rules to solve the problem:
| (-)-(-) = | (-)-(+) = | (+)-(-) = |
| (-15x)-(-5x) | (+12x)-(+8x) | (+5x)-(-6x ) |
| -15x+5x= -10 x | +12x-8x= +4x | +5x+6x= +11x |
Multiplication Operations Rules
When related words are combined to form an analogous statement of the multiplication, negative and negative generate positive values. Negative and positive terms multiply to generate a negative outcome, whereas positive and positive terms multiply to give a positive result. The following is an example of how to combine similar phrases using multiplication operations:
| (-)*(-) = | (-)*(+) = | (+)*(-) = | (+)*(+) |
| (-5)*(-5) =25 | (-5)*(+8)= -40 | (+5)*(-6 )=-30 | (+5)*(+7)=35 |
Division Operations Rules(/)
The division operations are applied in the same way as the multiplication operations were. The division of negative and negative yields positive values, but the division of negative and positive yields a negative result. The positive and positive phrases generate a positive outcome, thus combine them with division operations as follows:
| (-)/(-) = | (-)/(+) = | (+)*(-) = | (+)*(+) |
| (-10)/(-10) =+1 | (-10)/(+2)= -5 | (+15)*(-3 )=-5 | (+7)*(+7)=+1 |
You may use the verify all the calculations calculator by combining like phrases.
To discover the combining like terms solutions, we must first grasp how the combining equations calculator works. Let’s get started!
Input:
- In the input field, enter the coefficient, variables, and operators.
- You can enter fraction, monomial, polynomial, and exponential numbers, among others.
- Calculate by pressing the button.
Output:
The following computations are performed by the like terms combiner:
- The operation displays all phrases that are similar.
- All steps are depicted for our convenience.
- Click the recalculate button.
Combine Like terms calculator radical
When we are given particular values, we utilise the Radical Calculator to obtain the simplified radical expression. The radical of a number is also the number’s root (square root, cube root, or nth root).
Radical Calculator is a free online tool for simplifying radical expressions with the goal of eliminating the radical sign entirely if feasible. A radical expression is an algebraic expression made up of radicals.
To simplify a radical statement, we must take the nth root and simplify it. Assume our radical has the shape a n root over x. In this case, “a” represents the constant, “n” represents the nth root, and “x” represents the radicand or expression beneath the radical sign. To simplify a radical phrase, perform the methods outlined below:
- We begin by looking at the number under the radical sign. As a result, we shall first simplify n root over x.
- We’ll express x as a function of its prime components.
- Because we need to lower the nth root, we begin grouping the prime components with the same value in powers of n.
- The factors raised to the nth power can now be written outside of the radical sign. We delete the matching exponent after shifting.
- Multiply “a” by all of the components other than the radical sign.
- To obtain the simplified radical expression, multiply all remaining terms under the radical sign by a factor of two. If there are no terms under the radical sign, it is assumed that the radical sign has been removed.
Example 1
Simplify 4 3 roots over 135 and check it using an online radical calculator.
Solution:
We get the radicand as a product of its prime factors when we write it as a product of its prime factors.
4 3 roots over 135 = 4 3 roots over 3 into 3 into 3 into 3
= 4 into 3 into 3 root over 5
= 12 3 root over 5
As a result, 4 3 root over 135 may be decreased to 12 3 root over 5.
Example 2
Simplify 1.2 2 root over 144 and check it with an online radical calculator.
Solution:
We get the radicand as a product of its prime factors when we write it as a product of its prime factors.
1.2 2 root over 144 = 1.2 2 root over 2 into 2 into 2 into 2 into 3 into 3
Then, = 1.2 2 root over 2^2 into 2^2 into 3^2
= 1.2 into 2 into 2 into 3 into 2
= 14.4
Combine like terms calculator terms
Consider the formula 10×2 – 4×2, where the variables have the same exponent but different coefficients. We may reduce this formula even further by removing the identical variables from each other. This is achievable since the variables and exponents are the same regardless of the coefficients. The coefficients, together with the variables and exponent values, can be thought of as normal integers that stay constant after subtraction. As a result of reducing the formula, we obtain 10×2 – 4×2 = 6×2. Combining comparable phrases refers to the technique of simplifying the statement. The addition of comparable words is straightforward; for example, 5z + 12z + 32z = (5 + 12 + 32)z = 49z.

Unlike terms are ones whose variables and exponents vary. In an expression, if the coefficient is different, the variables are different (two variables), and the exponent powers are different, the expression is known to acquire, unlike terms. In contrast to algebraic terms, the algebraic statement 3x + 9y, where x and y are two separate variables with different coefficients, is known as.
Terms
Because the variables and exponents are not equivalent, simplifying formulas or combining like words cannot be done on unlike terms. For example, in 8xy + 6y – 9x – 10×2, there are several variables, exponents, and coefficients. This expression cannot be simplified since all of the words are distinct from one another.
- First, terms having the same exponents and variables.
- Then, variables and exponents with varying exponents
- Then, we can combine Similar terms to make them more concise.
- Also, combining dissimilar phrases does not simplify them.
- Also, we can add and subtract Similar phrases together.
- Then, we cannot add or subtract contrary words at the same time.
- Then, 13×2 + 5×2 is an example of a similar word.
- Also, 7z – 25r is an example of an opposite term.
- Similarly, like terms are also referred to as comparable terms.
- Atlast, unlike terms are sometimes referred to as dissimilar terms.
Some frequently asked questions
How do you combine like terms on a calculator?
This is a very basic tool for merging similar phrases. Please use the online combining like terms calculator to combine like terms of the algebraic statement as shown below:
- Navigate to any online combining like terms calculator.
- Enter the algebraic expression into the combining like terms calculator’s input box.
- To combine like phrases, click the “Solve” button.
- Click the “Reset” button to clear the field and start over.
Do you combine like terms or distribute first?
To begin, we distribute the constant terms into the terms enclosed by parenthesis. Then, rearrange the phrases such that they are grouped together. Finally, merge like phrases by adding or deleting as needed.
What is the difference between like term and unlike term?
- First, terms having the same exponents and variables.
- Then, variables and exponents with varying exponents
- Then, we can combine Similar terms to make them more concise.
- Also, combining dissimilar phrases does not simplify them.
- Also, we can add and subtract Similar phrases together.
- Then, we cannot add or subtract contrary words at the same time.
- Then, 13×2 + 5×2 is an example of a similar word.
- Also, 7z – 25r is an example of an opposite term.
- Similarly, like terms are also referred to as comparable terms.
- Atlast, unlike terms are sometimes referred to as dissimilar terms.
Can you combine XY and YX?
The variables’ order can be modified while being the same – that is, xy and yx are equivalent expressions, as are xy2 and y2x. Despite the fact that they are written in different orders, they have the same variables and exponents on those variables.
What is the combine like terms calculator?
The combine like terms calculator is a free online tool. Also, it can assist in combining like terms in an equation and simplifying it. Moreover, this is a useful tool for solving polynomial equation issues since it simplifies and speeds up the calculating process.
So, Combine like terms Calculator is an online app. Also, it allows you to combine like terms to simplify an algebraic statement. Then, we can see both like and unlike words in an algebraic expression. Then, the Combining Like Words Calculator assists you in combining like terms and reducing an algebraic statement. So, by adding or removing two similar words, they can be merged.
Is 3 x 3 a Monomial?
In algebra, a monomial is an expression that has just one term, such as 3xy. Monomials are made up of numbers, variables, or several numbers and/or variables multiplied together. Any single number, such as 5 or 2,700, is a monomial.
How do you multiply fractions?
When multiplying fractions, the first step is to multiply the two numerators. The denominators multiplied together in the second step. Finally, reduce the new fractions to their simplest form. Before multiplying, the fractions can be simplified by factoring out common factors in the numerator and denominator.
How do you solve double integrals on a calculator?
- Atfirst, open any Double Integral Calculator online.
- Then, fill in the function and the limitations in the provided input boxes. Choose which variable will be integratable first from the drop-down selection.
- Then, to obtain the value of the double integral, click the “Calculate” button.
- Atlast, select “Reset” to clear the fields and input new values.
How do you cross multiply on a calculator?
The Cross Multiplication Calculator is a free online calculator that displays the unknown number in a specified fraction. Any online cross multiplication calculator tool speeds up and simplifies computations. In a matter of seconds, the tool shows the unknown value.
The following is how to use the cross multiplication calculator:
- In each input field, enter the fractions with the unknown value “x.”
- To obtain the output, click the “Calculate x” button.
- In the output field “x,” the unknown value “x” will be printed.
What is an example of a combine like term?
Combine the similar terms 8×2 + 6x + 6×2 + 9 + 8x to simplify the following statement. Use the online combining like phrases calculator to confirm the answer.
Solution:
When we put similar phrases together, we get,
= 8 x 2 + 6 x 2 + 6 x + 8 x + 9
By combining similar phrases,
= 14×2 + 14x + 9
As a result, the sum of similar words Equals 14×2 + 14x + 9




